CABLES
SUBJECTED TO UNIFORMLY DISTRIBUTED LOAD
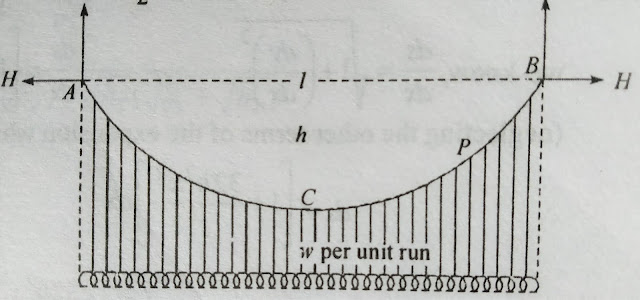
Consider
a cable of span L, subjected to uniformly distributed load of w/m throughout
the entire span. Let A and B are the two pinned supports which have the vertical
reactions Va and Vb, horizontal reaction H at both the ends.
Let h is the central dip (vertical distance) of the cable.
Due
to symmetry, the reactions Va and Vb are equal
Therefore,
Va = Vb = wL/2
Taking
moment about point C to determine the horizontal thrust
Va
(L/2) – H(h) -w(L/2) (L/4) = 0
(Note:
The value of the moment is taken as zero, since the cable structure will always
be free from moments)
(wL/2)
(L/2) – H(h) -w(L/2) (L/4) = 0
Solving
the above Eq
H = wL2/8h
Determination of tension forces
At
supports: Since, there are two forces in each support.i.e., one vertical
reaction and one horizontal reaction. Tension in the cable can be determined by
calculating the resultant of the above forces
Tension
at supports T = Resultant of V and H
T = √ (V2 +H2)
T = √ ((wL/2)2
+(wL2/8h)2)
Simplifying
the above equation,
Therefore
T = (wL/2) √ (1 +(L2/16h2))
Tension
at center span of the cable = The total shear force at that span from either
of the support
side
Shear
force due to vertical load V = Va – w(L/2)
= w(L/2) – w(L/2) = 0
Shear
force due to Horizontal thrust = - H (Since
it is in left direction hence taken
as negative)
= -wL2/8h
Total
tension force at the center = T = √ (V2 +H2)
T
= √ (02 +(-wL2/8h) 2)
Therefore,
Tension force at center = T = H = wL2/8h
Hence
by determining the tension at supports and center of span we can say that the
tension force is always maximum at supports and minimum at center.
Therefore,
Tmax = (wL/2) √ (1 +(L2/16h2))
Tmin = H = wL2/8h

Nice
ReplyDelete